

Designing Counterintuitive Structures via Optimization:
Mixed-Integer Programming Approaches
Dr. Yoshihiro Kanno
(Department of Mathematical Informatics, The University of Tokyo)
April 25 (Fri.), 2014 16:00-17:00
Seminar Room, 2nd floor, WPI-AIMR Main Bldg.
Materials with negative Poisson's ratio will expand transversely when stretched longitudinally. This property usually stems from a particular geometrical shape, e.g., a re-entrant structure. On the other hand, using two constituents with different positive thermal expansion coefficients, one can design three-phase materials, i.e., composites of two constituents combined with empty spaces, so as to have overall negative thermal expansion coefficients. Potential applications of those materials include fasteners, tunable filters, cushioning materials, and sensitive temperature sensors.
This talk presents optimization-based approaches to designing planar periodic frame structures that exhibit such counterintuitive properties. Specifically, the design problem is formulated as a mixed-integer linear programming problem, in which a linear objective function is minimized under the linear constraints and some binary constraints. The global optimal solution can then be found with an existing algorithm, e.g., a branch-and-bound method. Figure 1 shows an example of the obtained structures with negative Poisson’s ratio. Thus structural designs with unusual properties can be obtained without resorting to human intuition or experience. Also, the optimal solution has neither hinges nor thin members and satisfies local stress constraints.
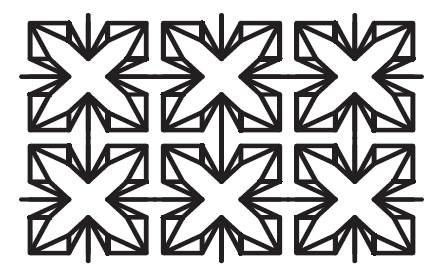
Figure 1: An obtained periodic frame structure that exhibits negative Poisson’s ratio.
General Affairs Section, AIMR Administrative Office
| TEL : | +81-22-217-5922 |
|---|---|
| E-MAIL : | wpi-soumu@wpi-aimr.tohoku.ac.jp |